

Should I treat my 10 Samples as a single one and use again this formula to find out? With this data I can compute the mean of all my 10 Samples means and this would be a good estimate of my population mean.īut how good is this new estimate when compared to the true population mean? Ok that's nice and good but let's say I have 10 indepentent Samples and in each I have 100 Observations. So F = MST/MSE.I know that the standard error of the sample mean estimates how good my sample mean is, compared to the population mean. Of course, you can’t calculate the SD with only one observations. 1) Because I am a novice when it comes to reporting the results of a linear mixed models analysis, how do I report the fixed effect, including including the estimate, confidence interval, and p. Then samples can be drawn from the estimated population and the sampling distribution of any type of. In addition, for cases where you don’t know the population standard deviation,, you can substitute it with s, the sample standard deviation from there you use a t-value instead of a z-value in your formulas as well. The idea is to use the observed sample to estimate the population distribution. Theoretically, SD SEM when you have a sample size of one. Bootstrap is a computer-based method for assigning measures of accuracy (bias, variance, confidence intervals, prediction error, etc.) to statistical estimates. This is the ratio of the two mean squares that we calculated. The trick to understanding the relationship between the standard deviation and SEM is that SEM has the SD in the numerator and the square root of the sample size in denominator. Calculate the mean square of treatment.The number of degrees of freedom of error is the total number of data points, minus the number of samples, or n - m. The number of degrees of freedom of treatment is one less than the number of samples used, or m - 1. The overall number of degrees of freedom is one less than the total number of data points in our sample, or n - 1. This section presents the standard errors of several random variables we have already seen: a draw from a box of numbered tickets, the sample sum and sample mean of n random draws with and without replacement from a box of tickets, binomial and hypergeometric random variables, geometric random variables, and negative binomial random variables.
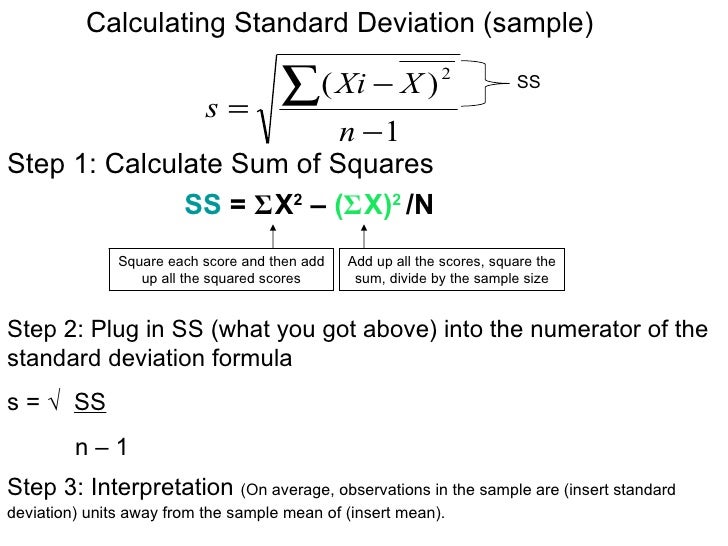
This number is the sum of squares of treatment, abbreviated SST. Finally, take the square root of that number to find the standard deviation. Next, add all the squared numbers together, and divide the sum by n minus 1, where n equals how many numbers are in your data set.
The sum of all of these squared deviations is multiplied by one less than the number of samples we have. Then, subtract the mean from all of the numbers in your data set, and square each of the differences. We square the deviation of each sample mean from the overall mean.


 0 kommentar(er)
0 kommentar(er)
